Radial and Spiral tilings

Steven Dutch, Professor Emeritus, Natural and Applied Sciences, University of Wisconsin - Green Bay

---

Radial tilings

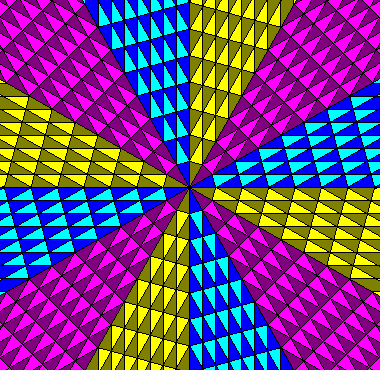Radial tilings are all ultimately based on sectors of tesselated triangles meeting at a central point.

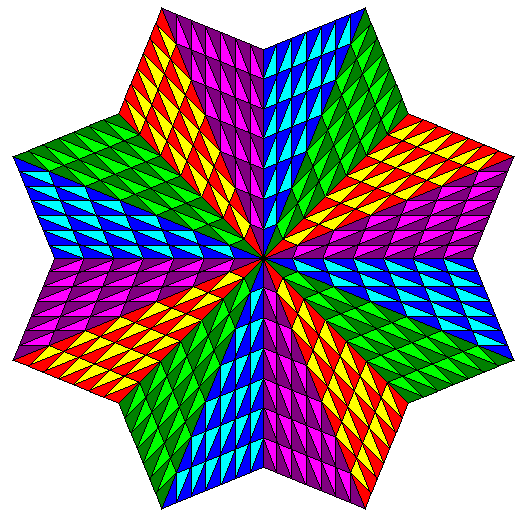
If the triangles are not isoceles, they can still form a radial tesselation with adjacent mirror-image sectors.

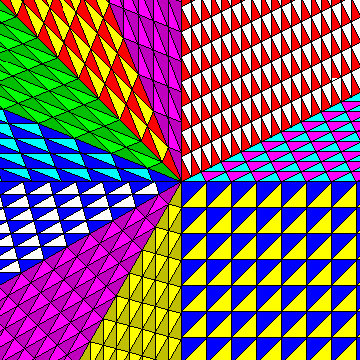	There is no rule that the wedges have to be equal in angle or that the triangles must meet edge-to-edge, but the resulting tesselations are not likely to be symmetrical or very attractive.
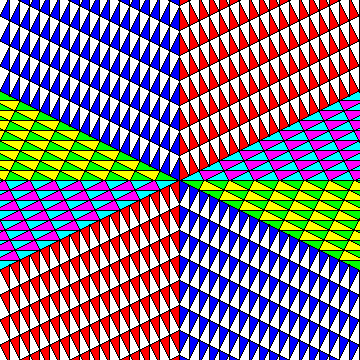	This tiling of 1 x 2 right triangles is not edge-to-edge or made of equal sectors but the rational proportions make it somewhat interesting.
	Radial tesselations can also be made up of figures created by joining or modifying triangles, in this case, to form rhombuses.

spiraltilings

	One type of spiral tiling is based on the fact that radial triangle tesselations with an even number of sectors are bisected by straight lines.
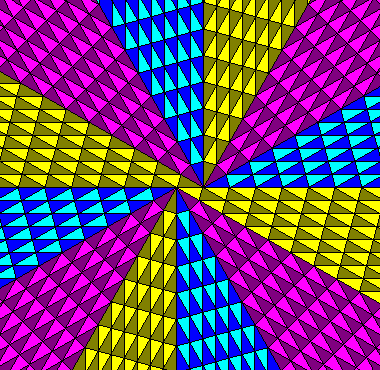	If we offset the two halves of a radial tiling along one of the straight lines, we obtain a spiral tiling. Note that the offset juxtaposes two sectors of identically-oriented triangles and creates a continuous "lane" of triangles.
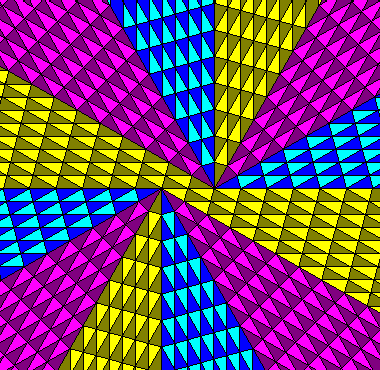	Here the tiling is offset by two cells. Note that the lane is wider. There is no way to eliminate it. If we remove it, we merely juxtapose two other sectors of identically-oriented triangles and create a new lane with different orientation.

	Spiral tilings are possible with non-isoceles tiles as well.
	We offset the two halves to obtain a spiral tiling with kinked spirals.
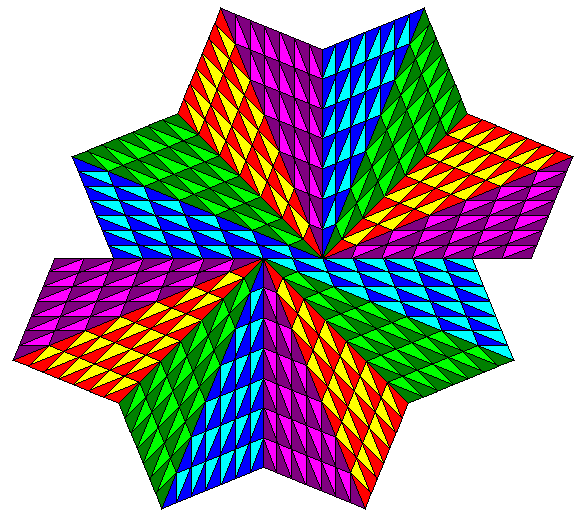	Here the tiling is offset by two cells.

	We can also form spiral tilings from other radial tesselations with an even number of sectors. Here we begin with a rhombus tiling.
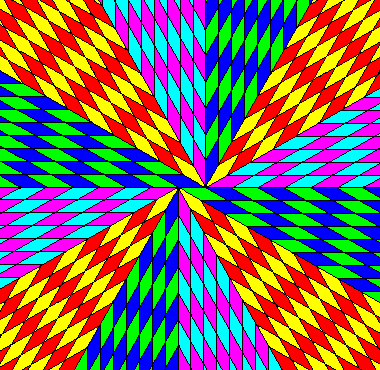	We offset the two halves of the rhombus tiling to obtain a spiral tiling. Note that the offset juxtaposes two sectors of identically-oriented rhombuses and creates a continuous "lane".
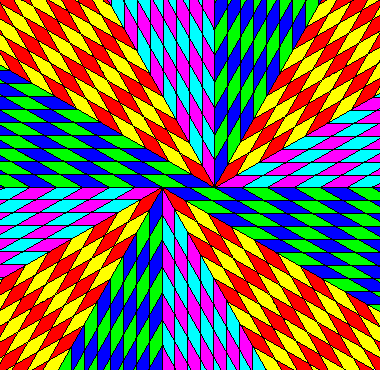	Here the tiling is offset by two cells.

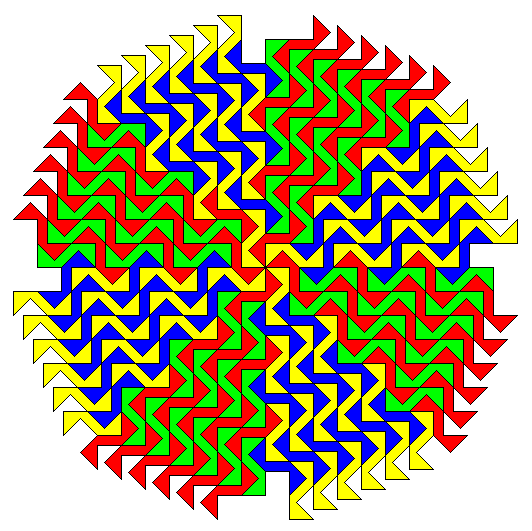This tesselation is made up of deformed 45 degree triangles. Because all the angles are 45 or 90 degrees, this tiling is especially well suited to computer graphics. Yellow and red tiles are mirror images of the green and blue tiles.

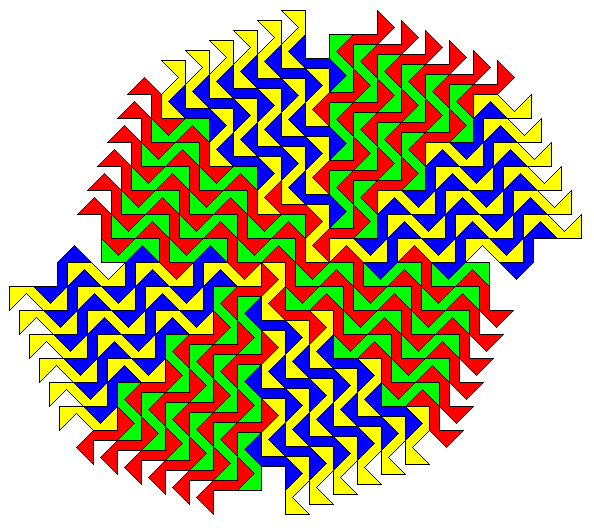Here the tiling has been offset by one tile.

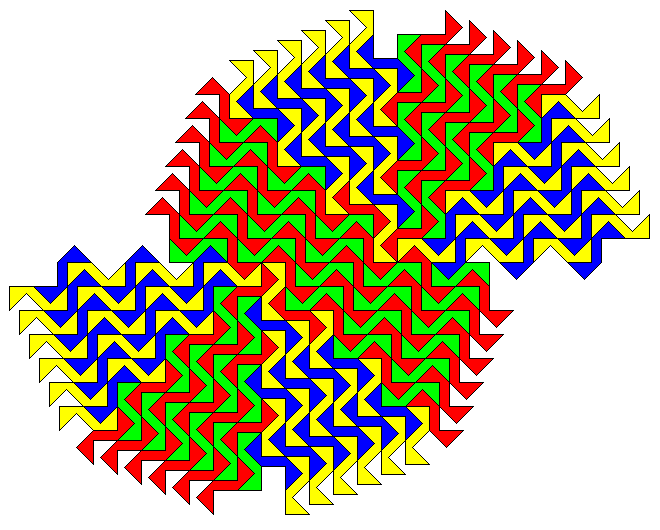Here the tiling has been offset by two tiles. Note again that the "lane" of identically oriented tiles appears.

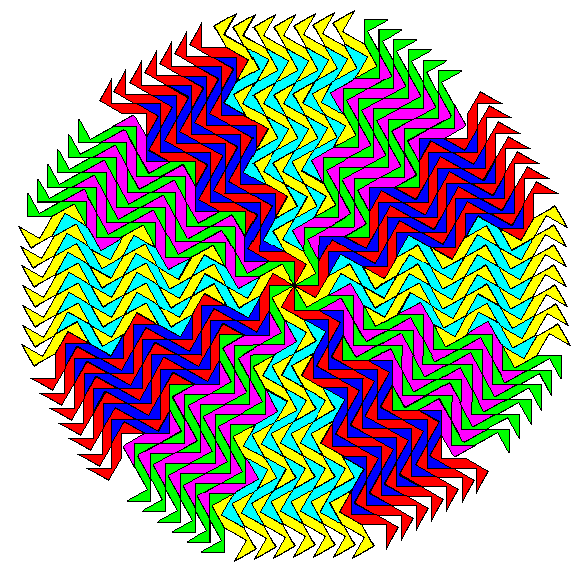This tiling is analogous to the one above except the tiles are based on 30-degree triangles.

Here the tiling has been offset by one tile.

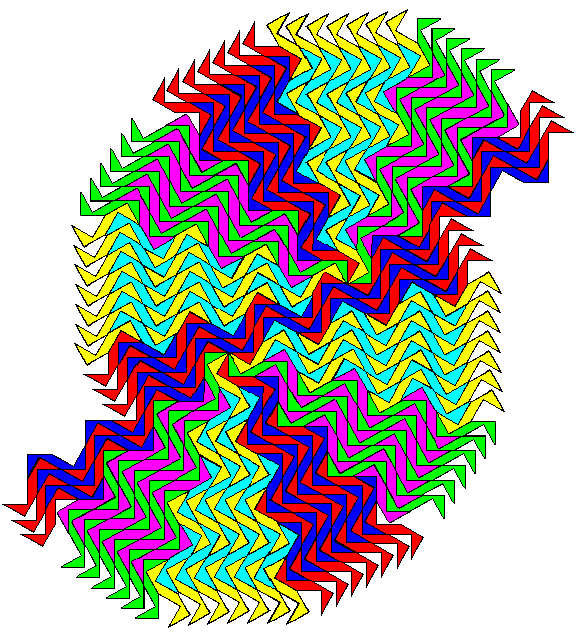Here the tiling has been offset by two tiles.

---

Some Special spiraltilings. View this page for additional spiral tilings.

Logarithmic spiraltilings. Logarithmic spirals lend themselves to beautiful tilings in which tiles change in size but are all geometrically similar. This property makes logarithmic spirals common growth patterns in biology.

---

Return to Symmetry Index
Return to Professor Dutch's home page

Created July 1, 1999, Last Update July 21, 1999